balijilo.blogg.se - Cinematica inversa de un robot scara

#Cinematica inversa de un robot scara how to

As we have x and y readily available, let’s choose the arctangent formula. Here, D1 can be calculated in two ways: The arcsine of y/dist or the arctangent of y/x.

In the following diagram, x, y, and dist define a right-angled triangle.

This comes handy in two places, as we’ll see shortly.įrom the robotic arm diagram above (the one with D1, D2, dist, etc), we can directly derive the first formula: A1 = D1 + D2ĭ1 is fairly easy to calculate. With this version, we can calculate angle C from the triangle’s sides a, b, and c. We do not need the basic form, but rather the transformed version that you can see below the original formula. The law of cosines (see the first formula in the figure above) is a generalization of the Pythagorean theorem (c 2 = a 2 + b 2 for right(-angled) triangles) to arbitrary triangles. Now is a good moment to dig out an old trig formula you may remember from school: The law of cosines. Furthermore, dist divides angle A1 into two angles D1 and D2. It points from (0,0) to (x,y), and as you can easily see, the three lines dist, len1, and len2 define a triangle. In the diagram you also see a new dotted line named dist.

The tip of segment 2 points to (x,y), and we want to calculate back from that point to the yet unknown values of A1 and A2.

The second joint describes an angle A2 measured from the first segment (counterclockwise in both cases).

The root joint describes an angle A1 measured from the x axis.

The segments have the length len1 and len2, respectively.

This diagram tells us a couple of things: Let me just tweak the diagram a little by replacing some of the labels and adding one line and two angles: Here is a schematic diagram of our robot:Īpplying the geometric approach to the SCARA robot Now you know why our robot just serves tea.) (Robot hands would have additional degrees of freedom, and remember that we want to keep things simple.

There is no hand attached to the end of the arm.

The axes of both joints have the same direction.

The segments can only rotate around their base joint there is no sliding movement.

The arm has only two segments of fixed length.

Our robotic arm shall meet the following requirements. So for this article, we’ll stick with what is probably the most simple robotic arm with rotary joints. (In a future article, I’ll give the numeric approach a try.)Īt this point, I must admit that when I started working on this article, I expected that the formulas for the simple two-segment arm could easily be generalized to multi-segment, multi-joint robotic arms, but I found that this is not the case. Luckily, all complexity vanishes in the case of a simple robotic arm with only two segments, so let’s go with this approach. The second one, the geometric approach, can become quite complex when the robot’s arm consists of many segments and joints. It involves a lot of matrix calculations, and frankly, I haven’t done any since the last millennium or so. Which one to pick? After all, each of them has its raison d’être.įor the sake of brevity, let’s drop the first one.

Move one or more segments to locally minimize the error. The numeric approach: Take a guess and look how far we are off.The geometric approach: The idea is to combine knowledge about the robotic arm’s geometry with suitable trigonometric formulas.The algebraic approach: This basically works by solving (frankly, rather complex) matrix equations.For inverse kinematics, there are three of them: And whenever something is hard to solve, there are usually several different approaches available for solving that problem.

#Cinematica inversa de un robot scara how to

This is quite the opposite of the previous calculation - here, we start with a given position and want to know how to rotate each segment of the arm. Now the robot’s arm must adjust each joint’s angle in order to move its hand over the cup.

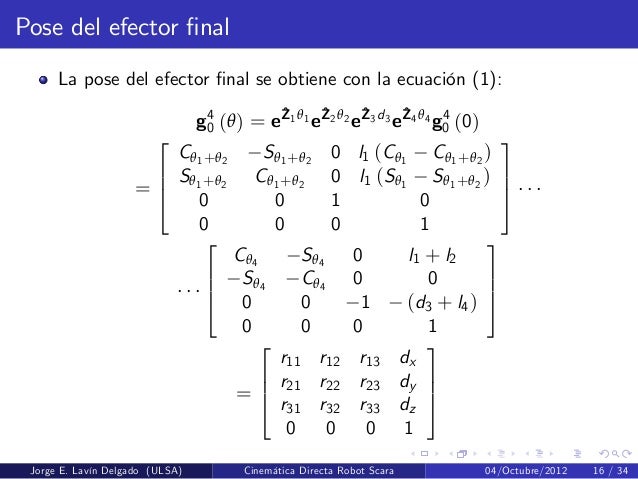
Et voilà: we determined the hand’s position. Repeat this with each segment, until we arrive at the robot’s hand. We just need to look at each segment of a robot’s arm–the coordinates of the segment’s base, the direction of the joint’s axis, the angle between this segment and the next one, and the length of the segment–in order to calculate where the end of this segment is. Calculating the current coordinates of a robot’s hand is easy.